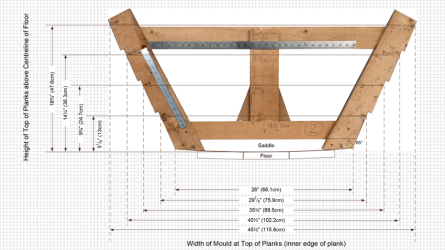
The Electric Launch Fusion and the Component Waveform Theory

FUSION, an electric day boat, is designed to a new theory describing how boats move through water. Her propulsion is an electric outboard motor, which rests in a lifting box and allows for silent cruising. FUSION’s retro-classic look is based upon the slipper launches of the Thames River. A hard-mounted sun awning provides protection from the harsh Australian sun.

For more than forty years, Alan Skinner has worked on a theory of boat design based upon the fact that a boat’s speed-robbing wavemaking resistance changes at different speeds. His theory involves designing for a boat’s optimal speed, using curves derived from the hull’s curve of Areas. In our most recent Sketchbook concept, Laurie McGowan designed the electric launch FUSION using Skinner’s theory—which he summarizes here.

The Component Waveform Theory

Alan Skinner has worked on his Component Waveform Theory for more than 40 years. The theory is contained in his booklet, Hull Speed, which also contains a summary of the evolution of boat design from ancient Phoenicia and Greece, to China, Micronesia and Polynesia, and Europe. It includes some very well-known names in science and hydrodynamics: Archimedes, Newton, Bernoulli, Chapman, Gerstner, Russell, Froude, Thomson (Lord Kelvin), among others.

Mathematicians first became involved in the theory of ship design in the early 1700s but by the latter half of the 1800s, tank-testing of scale models had proven to be a more reliable method to determine wave-making resistance. Lack of space doesn’t allow me to summarize well the evolution of design thinking and practice, but basically it came down to a battle of theoretical versus empirical. Today, we can test a model, change it a bit, and test again very quickly on a computer, based largely on extensive data derived from tank-testing. Then we can test an actual model if the expected results warrant the cost. Who needs theory?

In the beginning, Alan’s sailing of a very efficient open (with few rules) class of sailing dinghy, the Australian NS14, led him to wondering why some boats could move effortlessly from hull speed through the semi-planing to planing phases better than others. He developed first a way of understanding the forces on a boat in motion, then of optimizing the shape of the boat using the shapes of the waves that are formed, the boat’s waterline length and displacement, and the desired speed. At the heart of the theory is the understanding that wave-making is the biggest deterrent to speed and his thinking that the resultant wake is actually formed by an infinite number of low-amplitude “component” waves that are generated by a moving vessel.

He writes: “at each point in its path every surface vessel generates a circular component wave of unique proportions for each speed at which it travels and, being a rigid structure with a fixed waterline length and displacement, a hull cannot be designed to conform to the properties of those waves for any more than one particular speed. At all other speeds the hull’s proportions will be at variance with those of the component waves being generated, resulting in an increase in wave-making resistance and a hull design of less than maximum efficiency.” His words highlight how designing boats is a balance of trade-offs, but his target is to increase vessel efficiency by first picking a speed, then designing to the “correct” shape and distribution of hull volume.

In Hull Speed, Alan stresses from the outset that he is not qualified to put forward a theory on boat design, and although his Component Waveform Theory remains untested I find his logic convincing. Things finally clicked for me when I looked at his link between the immersed sections of the hull (as seen in the curve of areas) and the changing shape of the component wave for different boat speeds. He has a chart of curves of areas relating to speeds and calls these curves “the shape of the component wave,” but I’ll call them “component waveform (CW) curves” for simplicity. Although in Hull Speed Alan confined his logic to the performance of NS14s, in practice I think that you can specify the operating speed of whatever boat you’re working on, calculate how that speed relates to hull speed, then use the appropriate curve to shape the hull to. I used DWL=1.6 LWL (or CW curve 1.6) for FUSION, the concept boat I presented in my Sketchbook article in WoodenBoat No 261 (March/April 2018). You’ll still use common sense when designing, of course, as a boat with a dislplacement/length ratio of, say, 400 likely won’t be using a curve over hullspeed (or DWL = LWL in Fig. 1), and a double-ender, without planing sections, won’t be truly planing either.

How to use the CW Curves

Whether you’re designing a boat by hand or with software, you can draw your speed-defined CW Curve on the page, or import it as a background curve or image in your modelling or drafting program.

I surprised Alan by telling him I didn’t think the actual displacement of a boat being worked on or the height of the curve mattered, and that his curves for the NS14 might actually be universal, or almost universal. As with any curve in a graph, it’s the “shape,” or the relationship of one part to another of both the CW curve and curve of areas that matters, not their height.

With CAD software, you can highlight the whole curve of areas your software generates and stretch or squish it vertically very easily, then superimpose it over the CW curve you’re after. Each time you check the boat’s displacement and generate a curve of areas, you can compare it to the CW curve, then adjust the hull and try again.

As a vessel begins to plane, the more the transom is immersed. This means the bow rises, or has had a change of trim (usually defined as "positive"). It’s good to estimate this in the calculations when matching your final curve of areas to the CW curve.

Though it’s unproven, I find the Component Waveform Theory very appealing and have already started using it, and time will tell how well boats do that are designed using it.

If you’d like more information on the Component Waveform Theory, contact Alan Skinner at afshullspeed@gmail.com.

Laurie McGowan is a Nova Scotia–based boat designer with a diverse on- and below-water work history. He specializes in energy-efficient commercial and pleasure boats. More of his work may be found at mcgowanmarinedesign.com.

Designs: Sketchbook appears in every issue of WoodenBoat. McGowan takes readers’ ideas of their ideal boats and designs a vessel to those specifications. Do you have a boat concept you’d like to see Laurie McGowan develop? If so, send it to Sketchbook, WoodenBoat Publications, P.O. Box 78, Brooklin, ME 04616, or email it to sketchbook@woodenboat.com. Your letter should be no longer than 500 words.